If the law had an attic, it would hold the subject matter of Pool v. City of Houston, No. 19-20828 (Oct. 23, 2020):

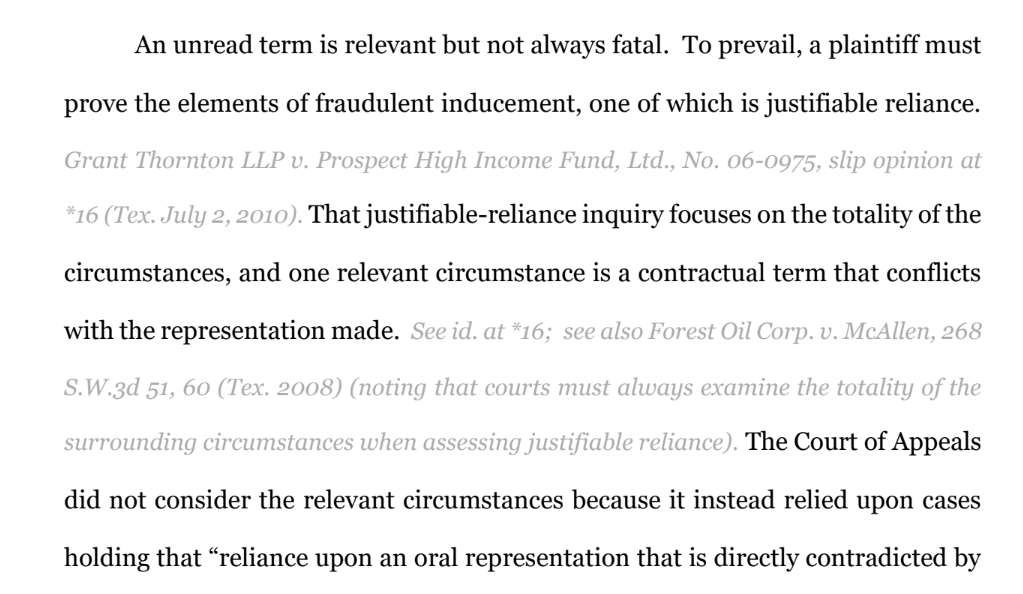
It is often said that courts “strike down” laws when ruling them unconstitutional. That’s not quite right. Courts hold laws unenforceable; they do not erase them. Many laws that are plainly unconstitutional remain on the statute books. Jim Crow-era segregation laws are one example. The City of Houston contends that it’s being sued for one of these so-called “zombie” laws. Its Charter allows only registered voters to circulate petitions for initiatives and referenda, even though the Supreme Court held a similar law unconstitutional twenty years ago. This case thus requires us to decide when the threat of continued enforcement is enough to reanimate a zombie law and bring it from the statutory graveyard into federal court.
 (citations omitted). Held: the zombie walked, based both on the City’s history of enforcement of this specific law, and the inadequacy of its effort to disclaim further enforcement: “At least based on the current record, the City’s addition of the ‘Editor’s note’ on its website does not moot this case. Voluntarily stopping an unconstitutional practice renders a case moot only ‘if subsequent events ma[k]e it absolutely clear that the allegedly wrongful behavior c[an] not reasonably be expected to recur.'” You can hear more about this case on the Coale Mind Halloween Special – “Attack of the Zombie Statute!”
(citations omitted). Held: the zombie walked, based both on the City’s history of enforcement of this specific law, and the inadequacy of its effort to disclaim further enforcement: “At least based on the current record, the City’s addition of the ‘Editor’s note’ on its website does not moot this case. Voluntarily stopping an unconstitutional practice renders a case moot only ‘if subsequent events ma[k]e it absolutely clear that the allegedly wrongful behavior c[an] not reasonably be expected to recur.'” You can hear more about this case on the Coale Mind Halloween Special – “Attack of the Zombie Statute!”